Identity Function

The identity function is a function that returns the same value that was used as its input.
Mathematically, it is defined as:

f(x) = x

It is also known as an identity relation, identity map, or identity transformation. This function maps each element in a set to itself, ensuring that the input and output are always identical.

Key Properties:

1. For all elements a ∈ A, if g (a) = a, then g(x) = x is called an identity function.
2. Every input corresponds to a unique and identical output.
3. The domain and range of an identity function are both the set of real numbers ℝ.
4. Every element in the domain is mapped to itself in the range.

Example:

If the input is 2, then the output is 2. If the input is 3, the output is 3. Representing it as a set of ordered pairs:

f = {(2, 2), (4, 4), (6, 6), (8, 8)}

Examples of Identity Function

Here are some functions that can be considered identity functions:

1) f: N → N, f(x) = √(x²)

For natural numbers( N), the function f(x) = √(x²) returns the absolute value of x which is "x" itself.

2) g: Z^- → Z^-, g(x) = -√(x²)

For negative integers (Z⁻), the function g(x) = −x² returns "x" unchanged but negated.

3) h: N → N, h(x) = |x|

For natural numbers, h(x) = ∣x∣ simply returns x since the absolute value of any natural number is the number itself.

4) k: Z^- → Z^-, k(x) = - |x|

For negative integers, k(x) = −∣x∣ returns x as it negates the absolute value of x, preserving the identity for negative values.

4) l: N → N, l(x) = ⌊x⌋

The function l(x) = ⌊x⌋, where ⌊x⌋ is the greatest integer function, also preserves the identity for natural numbers.

Note: All the functions which maps the same input to the same output are examples of identity functions.

Domain and Range of Identity Function

An identity function is a real-valued function that can be represented as f: R → R such that f(x) = x, for each x ∈ R. Here, R is a set of real numbers which is the domain and range of the function f. The domain and the range of identity functions are the same.

• Domain: The set of input values or x values of a function f(x).
  • The domain of the identity function f(x) is R

• Range: The set of output values or y values of a function f(x).
  • The range of identity function f(x) is R

Graphical Representation of Identity Function

To plot the graph of an identity function, start by taking the values of the x-coordinates on the x-axis and the y-coordinate values on the y-axis. The graph of an identity function is a straight line that passes through the origin. For an identity function, the domain and range are the same.

Consider an example for the identity function, f(x) = x,

Substitute x = -2, -1, 0, 1, 2 in f(x),

Plot these points and join these points on the graph as shown below,

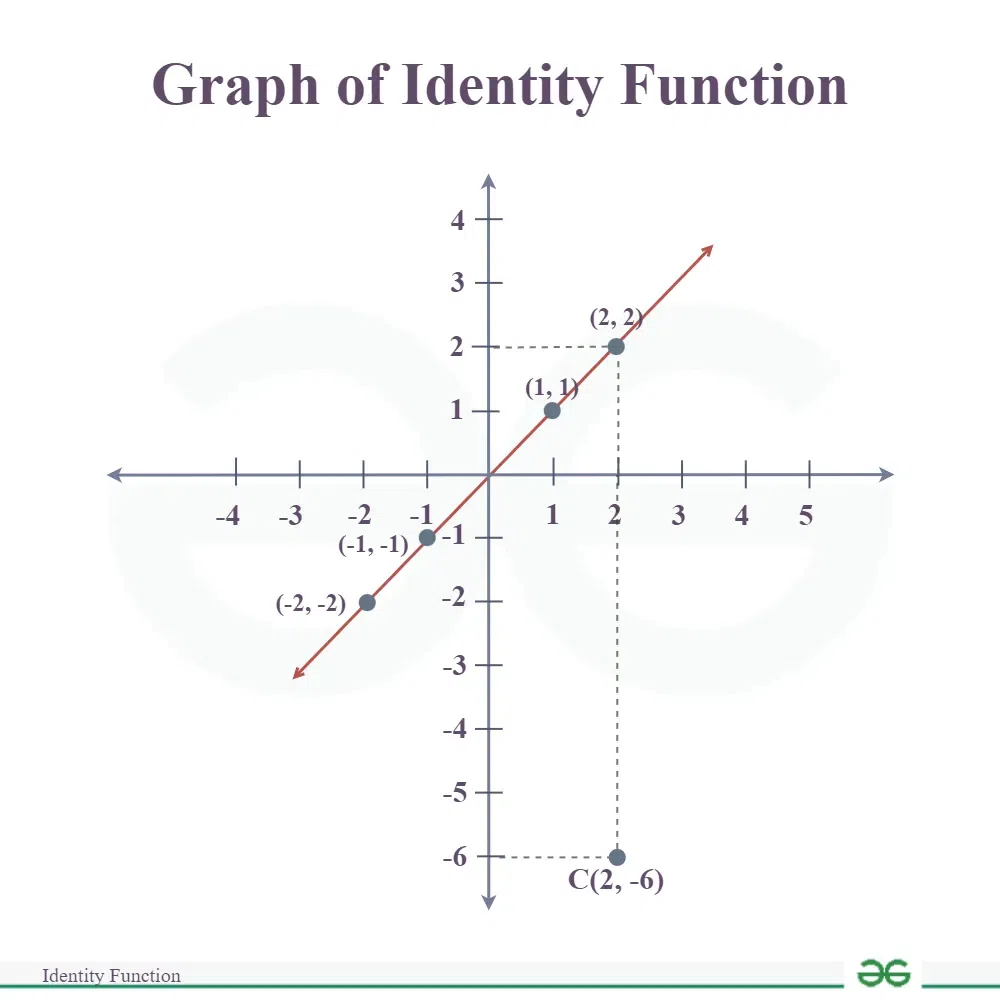
Slope and Equation of Graph of Identity Function

The graph of the identity function is a straight line line that makes a 45° angle with both the x-axis and y-axis.

The general slope-intercept form of the equation of a straight line is:

y = mx + c

Where:

• m is the slope
• c is y-intercept

Finding the Slope:

Consider two points on the identity function's graph:

• (x₁, y₁) = (-2, -2)
• (x₂, y₂ ) = (-1, -1)

The slope "m" is given is given by the formula:

m=\frac{y_2-y_1}{x_2-x_1}

Substitute the coordinates of the points:

⇒ m=\frac{-1-(-2)}{-1-(-2)}

⇒ m=\frac{1}{1}

⇒ m = 1

Thus, the slope m = 1.

Finding the y-intercept:

The y-intercept c is the value of y when x = 0. At this point, the graph intersects the y-axis, so:

• When x = 0, y = 0
• Hence, c = 0

Equation of the Graph:

Now, we write the equation of the identity function as: y = 1x + 0

simplifying, we get: y = x

Thus, the equation of the identity function is: y = x

Properties of Identity Function

Some key properties of the identity function:

• The identity function is a real-valued linear function.
• The domain and range of the identity function are the same.
• The graph of the identity function is a straight line that makes a 45° angle with both the x-axis and y-axis. The slope of the graph is always 1.
• The identity function is a one-to-one and onto function.
• Composing the identity function with itself results in the identity function, i.e. g ∘ g(y) = y.
• The inverse of the identity function is the identity function itself.

Derivative of Identity Function

Derivative of identity function is 1.

As derivative is defined as the rate of change of the function with respect to other variable or independent variable.

Let Identity function f(x) = x,

Use the derivative formula,

\frac{d}{dx}(x)=1

The derivative of Identity function f(x) with respect to 'x' is,

\frac{d}{dx}(f(x))=\frac{d}{dx}(x)=1

The derivative of the Identity function is '1'.

Integral of Identity Function

Integral of identity function is x²/2 + C.

The integral of a function is to find the area under the graph of a function for some interval.

Let Identity function f(x) = x,

Use the Integral formula,

\int x \ dx=\frac{x^2}{2}

The Integral of the Identity function f(x) is,

\int f(x) \ dx=\int x \ dx=\frac{x^2}{2} + C

Where C is the constant of integration.

How to Identify an Identity Function?

There are many ways to check whether or not any given function is identity or not. Some of these methods are:

• Compare the given function with the function definition.
• Plot the function on a graph.
• Substitute some values of x into the function and check if the output matches the input.

Let's consider an example for better understanding.

Example: Check whether f(x) = 2x is an identity function or not.

Solution:

Substitute x = 1 in f(x), we get
f(1)) = 2
As input is not same as output. Thus, f(x) = 2x isn't a identity function.

Read More,

• Function
• Types of Functions
• Invertible Functions
• Relations and Functions
• One-to-One Functions
• Composition of Functions
• Onto Functions (Surjective Functions)

Solved Examples of Identity Function

Example 1: Check whether f(x)=3x is an identity function or not.

Solution:

For the given function f(x)=3x,

Substitute different x values in f(x),

At x = -2
f(-2) = 3(-2) =-6

At x = -1
f(-1) = 3(-1) = -3

In table format is,

x	-2	-1	0	1	2
f(x) = 3x	-6	-3	0	3	6

As the output is not same as the input, f(x) = 3x is not an identity function.

Example 2: Check whether f(x) = √(x²) is an identity function or not.

Solution:

Substitute different x values in f(x),

At x = 1
f(1) = √(1²) = √1 = 1

At x = -1
f(-1) = √(-1²) = √1 = 1

At x = 2
f(2) = √(2²) = √4 = 2

At x = -2
f(-2) = √(-2²) = √4 = 2

In table format is,

x	-2	-1	0	1	2
√(x2)	2	1	0	1	2

As the output is not same as the input for negative numbers, the given function isn't an Identity function.

Note: But if we restrict the domain and range of this function to natural numbers. It is an example of the identity function.